
Back نجمة سداسية Arabic Хексаграм Bulgarian হেক্সাগ্রাম Bengali/Bangla Hexagrama Catalan Hexagram Czech Heksagram Danish Hexagramm German Εξάγραμμα (γεωμετρία) Greek Hexagrama Spanish Heksagramm Estonian
This article needs additional citations for verification. (March 2023) |
| Regular hexagram | |
|---|---|
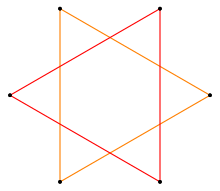 A regular hexagram | |
| Type | Regular polygonal figure |
| Edges and vertices | 6 |
| Schläfli symbol | a{6}, {6/2}, 2{3} or {{3}} |
| Coxeter–Dynkin diagrams | |
| Symmetry group | Dihedral (D6) |
| Internal angle (degrees) | 60° |
| Properties | star, compound, cyclic, equilateral, isogonal, isotoxal |
| Dual polygon | self |
| Star polygons |
|---|
|
|

A hexagram (Greek) or sexagram (Latin) is a six-pointed geometric star figure with the Schläfli symbol {6/2}, 2{3}, or {{3}}. Since there are no true regular continuous hexagrams, the term is instead used to refer to a compound figure of two equilateral triangles. The intersection is a regular hexagon.
The hexagram is part of an infinite series of shapes which are compounds of two n-dimensional simplices. In three dimensions, the analogous compound is the stellated octahedron, and in four dimensions the compound of two 5-cells is obtained.
It has been historically used in various religious and cultural contexts and as decorative motifs. The symbol was used as a decorative motif in medieval Christian churches and Jewish synagogues.[1] In the medieval period, a Muslim mystical symbol known as the Seal of Solomon was depicted as either a hexagram or pentagram.[2][3]
- ^ Scholem 1949, p. 244:"It is not to be found at all in medieval synagogues or on medieval ceremonial objects, although it has been found in quite a number of medieval Christian churches again, not as a Christian symbol but only as a decorative motif. The appearance of the symbol in Christian churches long before its appearance in our synagogues should warn the overzealous interpreters. "
- ^ Scholem 1949, p. 246:"In the beginning these designs had no special names or terms, and it is only in the Middle Ages that definite names began to be given to some of those most widely used. There is very little doubt that terms like these first became popular among the Muslims, who showed a tremendous interest in all the occult sciences, arranging and ordering them systematically long before the Practical Cabalists thought of doing so.It is not to be wondered at, therefore, that for a long time both the five-pointed and the six-pointed stars were called by one name, the "Seal of Solomon," and that no distinction was made between them. This name is obviously related to the Jewish legend of Solomon's dominion over the spirits, and of his ring with the Ineffable Name engraved on it. These legends expanded and proliferated in a marked fashion during the Middle Ages, among Jews and Muslims alike, but the name, "Seal of Solomon," apparently originated with the Muslims."
- ^ Leonora Leet, "The Hexagram and Hebraic Sacred Science" in :The Secret Doctrine of the Kabbalah, 1999, pp. 212-217.